Capacitor

A capacitor is circuit element which is capable of storing and delivering finite amount of charges. A capacitor essentially consists of two conducting surfaces (plates) separated by a layer of insulating medium called dielectric. One plate of the capacitor (say A) is positively charged, while the other (say B) has negative charge. The charge stored in a capacitor is proportional to the potential difference between the two plates.

When a capacitor is put across a battery there is momentary flow of electrons from A to B. Hence, a potential difference is established between plates A and B. This transient flow of electron gives rise to charge in current. As the charge on plates goes on increasing the voltage across the capacitor goes on increasing. After sometime the voltage across the capacitor becomes equal to voltage of dc source and charging currents’ i’ becomes zero. If dc source is removed now, the capacitor remains charged if such a charged capacitor is shunted by a resistance it will get discharge and voltage across it becomes zero after some time.

Mathematically, capacitance of capacitor is defined as the amount of charge required to create potential difference (PD) of 1 volt.

i.e.  C = Q/V

Ø  Q = CV

The capacitance is a measure of the amount of charge a capacitor can store; this is determined by the capacitor geometry and by the kind of dielectric between the plates. For a parallel plate capacitor made up of two plates of area A and separated by a distance d, with dielectric material, the capacitance is given by:

C = ε A / d   where ε = εr * εo  is permittivity of the dielectric material, εr is the relative permittivity and εo = 8.85x10־¹² F/m or C²/Nm²  is the permittivity of the free space. With dielectric material, the capacitance is given by:

C = εo A/d

Note that capacitance has units of farads (F). A 1 F capacitor is exceptionally large; typical capacitors have capacitances in the pF - microfarad range.

Dielectrics, insulating materials placed between the plates of a capacitor, cause the electric field inside the capacitor to be reduced for the same amount of charge on the plates. This is because the molecules of the dielectric material get polarized in the field, and they align themselves in a way that sets up another field inside the dielectric opposite to the field from the capacitor plates. The dielectric constant is the ratio of the electric field without the dielectric to the field with the dielectric:

Note that for a set of parallel plates, the electric field between the plates is related to the potential difference by the equation:

for a parallel-plate capacitor: E = V / d

For a given potential difference (i.e., for a given voltage), the higher the dielectric constant, the more charge can be stored in the capacitor. For a parallel-plate capacitor with a dielectric between the plates, the capacitance is:

Energy stored in a capacitor

The energy stored in a capacitor is the same as the work needed to build up the charge on the plates. As the charge increases, the harder it is to add more. Potential energy is the charge multiplied by the potential, and as the charge builds up the potential does too. If the potential difference between the two plates is V at the end of the process, and 0 at the start, the average potential is V / 2. Multiplying this average potential by the charge gives the potential energy : PE = 1/2 Q V.

Substituting in for Q, Q = CV, gives:

The energy stored in a capacitor is: U = 1/2 C V²

Capacitors have a variety of uses because there are many applications that involve storing charge. A good example is computer memory, but capacitors are found in all sorts of electrical circuits, and are often used to minimize voltage fluctuations. Another application is a flash bulb for a camera, which requires a lot of charge to be transferred in a short time. Batteries are good at providing a small amount of charge for a long time, so charge is transferred slowly from a battery to a capacitor. The capacitor is discharged quickly through a flash bulb, lighting the bulb brightly for a short time.

If the distance between the plates of a capacitor is changed, the capacitance is changed. For a charged capacitor, a change in capacitance correspond to a change in voltage, which is easily measured. This is exploited in applications ranging from certain microphones to the the keys in some computer keyboards.

Playing with a capacitor

To help understand how a capacitor works, we can experiment using a power supply, a capacitor, and a piece of dielectric material. The power supply provides the voltage, or potential difference, that causes charge to build up on the capacitor plates.

With the power supply connected to the capacitor, a constant difference in potential is maintained between the two plates. This results in a certain amount of charge moving on to the plates from the power supply, and there is a particular electric field between the plates. When some dielectric material is inserted between the plates, the field can not change because the potential difference is constant, and E = V / d. To ensure that the field does not change, charge flows from the power supply to the plates of the capacitor. Removing the dielectric causes the charge to flow back to the power supply, keeping the field constant. To summarize, when the voltage is fixed but the capacitance changes, the amount of charge on the plates changes.

On the other hand, if the power supply is connected to the capacitor briefly and then removed, it will be the charge that stays constant. If a dielectric material is inserted between the plates in this case, the field between the plates will be reduced, as will the potential difference. Removing the dielectric increases the field, and therefore increases the voltage.

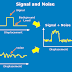
Electric fields and potentials in the human body

The body is full of electrical impulses, and we can measure these signals using electrodes placed on the skin. The rhythmic contractions of the heart, for example, are caused by carefully timed electrical impulses. These can be measured with an electrocardiogram (ECG or EKG). If the heart is malfunctioning, this will usually produce a change in the electrical activity of the heart, with particular changes corresponding to particular problems. Similar analysis can be done on the brain using an electroencephalogram (EEG).

Click on EKG's and heart arrythmias for a look at how heart arythmias are investigated using EKG's, as well as for a look at a typical electrical signal from a normal heart.