Filter

Filter can be considered can be considered as recurrence specific systems. A channel is required to isolate an undesirable flag from a blend of needed and undesirable signs. The channel determination are by and large given as far as cutoff frequencies, pass band (P.B) and stop band (s.b) districts. P. B is the recurrence band of needed flag and S.B is the recurrence band of undesirable flag. A perfect channel should pass the needed flag with no lessening and give endless weakening. Contingent on the segments utilized, channels can be delegated: 1. latent channels: Filters which are the compotnet, for example, R,L,C are the uninvolved channels. The Gains of such channels are in every case not exactly or equivalent to solidarity (i.e GS1). It is to be noticed the L and C are channel parts, yet R isn't. 2. Dynamic channels: The channels which utilize the parts, for example, transistors, operation amp and so forth are the dynamic channels. The Gains of such channels are constantly more prominent than or equivalent to solidarity. ( G ≥ 1)

Gain and Attenuation:   

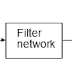
Let us consider the filters network with i/p V1(t)  having power P1 and o/p V2(t) having power p2 as shown in fig1. Then the transfer function is given by T(s) = V2(s)/V1(s) Where , V1(s) and V2(s) are the Laplace Transform of V1(t) . 

Then the voltage gain in db is given by ,  Av = 20log10 |T(jw )|  dB …………….(1) 

Or in term of power , the power gain is given by,  

Now, the voltage attenuation is given by ,  α = 1/Av    α = -20log|T(jw )| dB…………….(2) 

From equation 1 and 2 ,we can write,  

Types of filters: ( According to the function)   

Filters are classified according to the functions they are to perform. The pattern of PB and SB that give rise to the most common filters as defined below:

1. Low pass filters: (LPF):  A LPF characteristics is one in which the PB extend from ω = 0 to ω = ωc where ωc is know as cut off frequency. 

2. High pass filter:  A  high pass filter is a complement of a low pass filter in that the frequency range form o to ωc is the SB and from ωc to infinity  is the PB. 

3. Band pass filter ( BPF):  A BPF is one in which the frequency extending form ωL (or ω1) to ωu (ω2 ) are passed while signals at all other frequencies are stopped. 

4. Band stop filter(BSF):  A BSF is complement of BPF where signal components at frequencies form ω1 to ω2 are stopped and all others are passed. These filters are sometimes known as “Notch filters”. 

5. All pass filters (APF): It is a filter which passes all range of frequencies , i.e , PB ranges from o to infinity. 

Non- ideal Characteristics: 

         Filter                          Gain curve                                      Attenuation curve 

1. From the attenuation curve it to be noted that in the pass band the attenuation is always less then a maximum value. Designated as αmax
2. In the stop band the attenuation is always larger then a minimum value designated as α min .
3. Band between PB and SB so defined are known as transition bands. (TB).